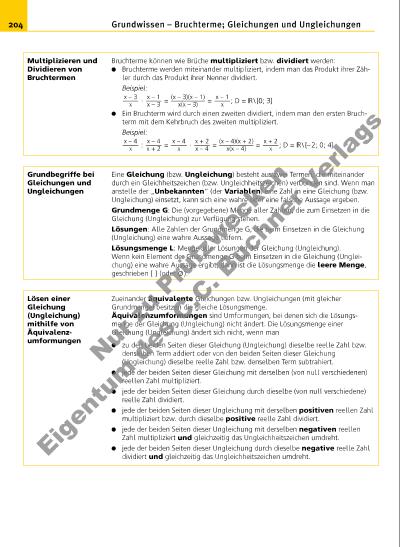
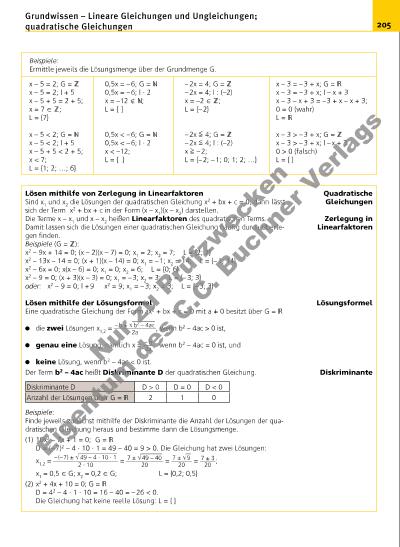
205 Lösen mithilfe von Zerlegung in Linearfaktoren Sind x1 und x2 die Lösungen der quadratischen Gleichung x 2 + bx + c = 0, dann lässt sich der Term x2 + bx + c in der Form (x – x1)(x – x2) darstellen. Die Terme x – x1 und x – x2 heißen Linearfaktoren des quadratischen Terms. Damit lassen sich die Lösungen einer quadratischen Gleichung häufi g durch Überlegen fi nden. Beispiele (G = ): x2 – 9x + 14 = 0; (x – 2)(x – 7) = 0; x1 = 2; x2 = 7; L = {2; 7} x2 – 13x – 14 = 0; (x + 1)(x – 14) = 0; x1 = – 1; x2 = 14; L = {– 1; 14} x2 – 6x = 0; x(x – 6) = 0; x1 = 0; x2 = 6; L = {0; 6} x2 – 9 = 0; (x + 3)(x – 3) = 0; x1 = – 3; x2 = 3; L = {– 3; 3} oder: x2 – 9 = 0; | + 9 x2 = 9; x1 = – 3; x2 = 3; L = {– 3; 3} Lösen mithilfe der Lösungsformel Eine quadratische Gleichung der Form ax2 + bx + c = 0 mit a 0 besitzt über G = die zwei Lösungen x1,2 = – b ± √ _______ b2 – 4ac ____________ 2a , wenn b2 – 4ac > 0 ist, genau eine Lösung, nämlich x = – b ___ 2a , wenn b2 – 4ac = 0 ist, und keine Lösung, wenn b2 – 4ac < 0 ist. Der Term b2 – 4ac heißt Diskriminante D der quadratischen Gleichung. Beispiele: Finde jeweils zunächst mithilfe der Diskriminante die Anzahl der Lösungen der quadratischen Gleichung heraus und bestimme dann die Lösungsmenge. (1) 10x2 – 7x + 1 = 0; G = D = (– 7)2 – 4 · 10 · 1 = 49 – 40 = 9 > 0. Die Gleichung hat zwei Lösungen: x1,2 = – (– 7) ± √ ____________ 49 – 4 · 10 · 1 ___________________ 2 · 10 = 7 ± √ _______ 49 – 40 ___________ 20 = 7 ± √ __ 9 ______ 20 = 7 ± 3 _____ 20 ; x1 = 0,5 X G; x2 = 0,2 X G; L = {0,2; 0,5} (2) x2 + 4x + 10 = 0; G = D = 42 – 4 · 1 · 10 = 16 – 40 = – 26 < 0. Die Gleichung hat keine reelle Lösung: L = { } Quadratische Gleichungen Zerlegung in Linearfaktoren Lösungsformel Diskriminante Beispiele: Ermittle jeweils die Lösungsmenge über der Grundmenge G. x – 5 = 2; G = x – 5 = 2; | + 5 x – 5 + 5 = 2 + 5; x = 7 X ; L = {7} x – 5 < 2; G = x – 5 < 2; | + 5 x – 5 + 5 < 2 + 5; x < 7; L = {1; 2; …; 6} 0,5x = – 6; G = 0,5x = – 6; | · 2 x = –12 x ; L = { } 0,5x < – 6; G = 0,5x < – 6; | · 2 x < –12; L = { } – 2x = 4; G = – 2x = 4; | : (–2) x = –2 X ; L = {– 2} – 2x 4; G = – 2x 4; | : (–2) x – 2; L = {– 2; – 1; 0; 1; 2; …} x – 3 = – 3 + x; G = x – 3 = – 3 + x; | – x + 3 x – 3 – x + 3 = – 3 + x – x + 3; 0 = 0 (wahr) L = x – 3 > – 3 + x; G = x – 3 > – 3 + x; | – x + 3 0 > 0 (falsch) L = { } Diskriminante D D > 0 D = 0 D < 0 Anzahl der Lösungen über G = 2 1 0 Grundwissen – Lineare Gleichungen und Ungleichungen; quadratische Gleichungen Nu r z u Pr üf zw ec ke n Ei ge nt um d es C .C . B uc hn er V er la gs