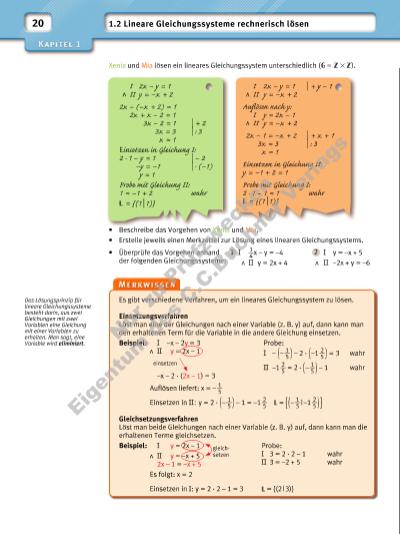
| Volltext anzeigen | |
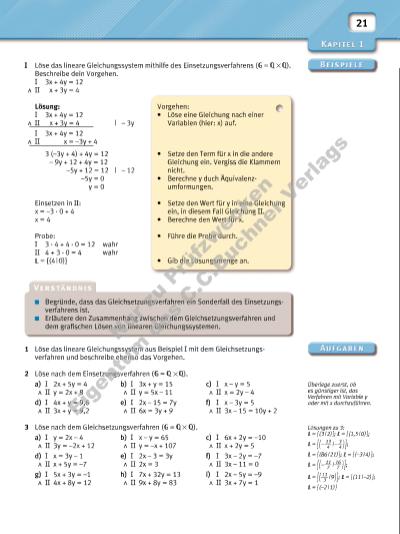
21 I Löse das lineare Gleichungssystem mithilfe des Einsetzungsverfahrens ( = ). Beschreibe dein Vorgehen. I 3x + 4y = 12 II x + 3y = 4 Lösung: I 3x + 4y = 12 II x + 3y = 4 | – 3y I 3x + 4y = 12 II x = –3y + 4 3 (–3y + 4) + 4y = 12 – 9y + 12 + 4y = 12 –5y + 12 = 12 | – 12 –5y = 0 y = 0 Einsetzen in II: x = –3 · 0 + 4 x = 4 Probe: I 3 · 4 + 4 · 0 = 12 wahr II 4 + 3 · 0 = 4 wahr = {(4 | 0)} 1 Löse das lineare Gleichungssystem aus Beispiel I mit dem Gleichsetzungsverfahren und beschreibe ebenso das Vorgehen. 2 Löse nach dem Einsetzungsverfahren ( = ). a) I 2x + 5y = 4 b) I 3x + y = 15 c) I x – y = 5 II y = 2x + 8 II y = 5x – 11 II x = 2y – 4 d) I 4x + y = 9,6 e) I 2x – 15 = 7y f) I x – 3y = 5 II 3x + y = 9,2 II 6x = 3y + 9 II 3x – 15 = 10y + 2 3 Löse nach dem Gleichsetzungsverfahren ( = ). a) I y = 2x – 4 b) I x – y = 65 c) I 6x + 2y = –10 II 3y = –2x + 12 II y = –x + 107 II x + 2y = 5 d) I x = 3y – 1 e) I 2x – 3 = 3y f) I 3x – 2y = –7 II x + 5y = –7 II 2x = 3 II 3x – 11 = 0 g) I 5x + 3y = –1 h) I 7x + 32y = 13 i) I 2x – 5y = –9 II 4x + 8y = 12 II 9x + 8y = 83 II 3x + 7y = 1 Begründe, dass das Gleichsetzungsverfahren ein Sonderfall des Einsetzungsverfahrens ist. Erläutere den Zusammenhang zwischen dem Gleichsetzungsverfahren und dem grafi schen Lösen von linearen Gleichungssystemen. Überlege zuerst, ob es günstiger ist, das Verfahren mit Variable y oder mit x durchzuführen. Vorgehen: • Löse eine Gleichung nach einer Variablen (hier: x) auf. • Setze den Term für x in die andere Gleichung ein. Vergiss die Klammern nicht. • Berechne y duch Äquivalenzumformungen. • Setze den Wert für y in eine Gleichung ein, in diesem Fall Gleichung II. • Berechne den Wert für x. • Führe die Probe durch. • Gib die Lösungsmenge an. Lösungen zu 3: = { (3 | 2) } ; = { (1,5 | 0) } ; = { ( – 13 ___ 4 | – 3 __ 4 ) } ; = { (86 | 21) } ; = { (–3 | 4) } ; = { ( – 11 ___ 7 | 16 ___ 7 ) } ; = { ( 11 ___ 3 | 9 ) } ; = { (11 | –2) } ; = { (–2 | 1) } Nu r z u Pr üf zw ec ke n Ei ge nt um d es C .C .B uc hn er V er la gs | |
 « |  » |
|
» Zur Flash-Version des Livebooks | |