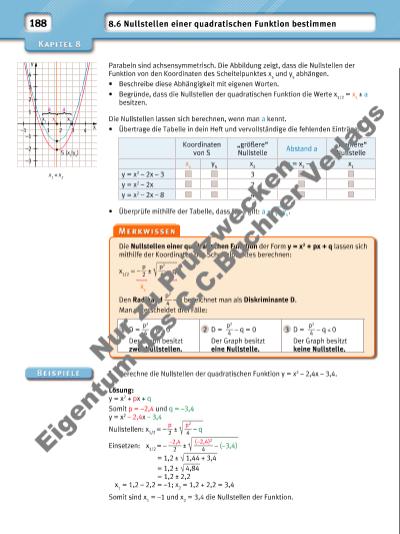
189 II Ermittle die Anzahl der Nullstellen der quadratischen Funktion. Bestimme anschließend die Nullstellen. a) y = x2 – 6x – 7 b) y = x2 – 8x + 25 c) y = x2 + 2x + 1 Lösung: Anzahl der Nullstellen mit der Diskriminante D = p2 __ 4 – q bestimmen: a) p = –6; q = –7 b) p = –8; q = 25 c) p = 2; q = 1 D = (–6)2 ____ 4 – (–7) D = (–8)2 ____ 4 – 25 D = 22 __ 4 – 1 D = 16 D = – 9 D = 0 D 0: D 0: D = 0: Der Graph besitzt Der Graph besitzt Der Graph besitzt zwei Nullstellen. keine Nullstelle. eine Nullstelle. x1/2 = – –6 ___ 2 ± √ ___ 16 x1/2 = – 2 __ 2 ± √ __ 0 x1 = –1; x2 = 7 x1 = –1 Begründe, dass es geschickt ist, zuerst die Diskriminante zu bestimmen und erst dann mögliche Nullstellen zu berechnen. Sophia behauptet: „Um die Anzahl der Nullstellen zu ermitteln, muss ich nicht rechnen, wenn ich den Scheitelpunkt einer quadratischen Funktion kenne.“ Erkläre diese Behauptung. 1 Ermittle die Koeffi zienten p und q. Bestimme die Anzahl der Nullstellen. a) y = x2 + 18x – 24 b) y = x2 – 1 __ 8 x + 2 1 __ 5 c) y = x 2 – 17x + 13 d) y = x2 + x – 3,5 e) y = x2 – 2,9x + 8,5 f) y = x2 + 1 __ 5 x – 17 ___ 24 2 Berechne die Nullstellen der Funktion. a) y = x2 + 4x + 3 b) y = x2 – 16x – 17 c) y = x2 – 20x + 36 d) y = x2 + 5x – 2,75 e) y = x2 – 3x – 1,75 f) y = x2 + x – 3 __ 4 3 Berechne die Nullstellen. Runde sinnvoll. a) y = x2 + 7x – 2,5 b) y = x2 – 4 __ 5 x – 3 3 __ 4 c) y = x 2 + 17x + 13 d) y = x2 + 2,5x – 12,75 e) y = x2 – 5,8x – 4,7 f) y = x2 + 5 __ 7 x – 13 ___ 14 4 Zeichne den Graph der Funktion und lies die Nullstellen so genau wie möglich ab. Nutze den Scheitelpunkt und die Schablone der Normalparabel. a) y = x2 – 4x + 3 b) y = x2 – 5x + 2,25 c) y = x2 – 8x + 7 d) y = x2 + 10x + 25 e) y = x2 – 3x + 1,25 f) y = x2 + 3 __ 2 x + 9 ___ 16 g) y = x2 – 1 __ 2 x – 2,5 h) y = x 2 – 4x – 1,5 i) y = x2 – 4 __ 3 x – 2 5 Bestimme die Anzahl der Nullstellen mithilfe der Diskriminante D. a) y = x2 + 8x + 16 b) y = x2 – 2x + 2 c) y = x2 + 17x + 13 d) y = x2 + 4x + 10 e) y = x2 – 6x + 9,5 f) y = x2 – 9x g) y = x2 – 2x – 5 h) y = x2 + 1 __ 2 x – 3 __ 4 i) y = x 2 – 4,5x + 7,5 x1/2 = – p __ 2 ± √ __ D Lösungen zu 2: 0,5; 18; –1; –3; –0,5; –1; 2; –5,5; – 3 __ 2 ; 3,5; 1 __ 2 , 17 Lösungen zu 4: –5; –1,35; –0,9; –0,75; –0,35; 0,5; 0,5; 1; 1; 1,85; 2,23; 2,5; 3; 4,35; 4,5; 7 Nu r z u Pr üf zw ec ke n Ei ge nt um d es C .C .B uc hn er V er la gs